There is a problem in how we teach:
We typically show pupils only the classic forms of a problem or a procedure.
It’s easily explained: time and fear. It takes ages to show lots of examples, and it’s also scary. They might be bored. They might not get it. When we want to be kind to ourselves, I think we say we don’t want to spoon-feed, but I suspect it is also due to thinking only about the specific questions we want them to be able to answer. It’s goal-focused thinking (typically good), but the goal is too narrow (that lesson’s questions). The upshot is that they can’t generalise…the most prized of mathematical skills. I’m tentatively concluding that this is a huge problem.
I suspect that, even if we fixed behaviour, bad leadership, too little contact time, and all the other things that can impede learning, this problem would still cause the long tail of underachievement we see across the country. I suspect it explains why pupils with anything less than an A* are bewildered by A-level maths (and even those pupils look pretty shocked), whereas pupils with As and Bs can cope in other courses.
We suffer from three problems, as maths teachers:
- expert-induced blindness (we can’t see what we can’t see because we can’t remember finding it difficult to understand)
- We can see the connections and logical conclusions in maths, and it is obvious how things generalise. We don’t even notice ourselves generalising.
- Algebraic reasoning is really hard to teach. People who can reason algebraically feel like they are applying common sense. I suspect a more accurate description of is “a deep understanding of the order of operations (and willingness to test by substituting)”
It took me a while to realise why I have decent algebraic reasoning. I struggled a lot at A-level (which is why I like teaching maths, incidentally). Everything else before that seemed easy. As in, I filled time after finishing exercises with finding new ways to do them (e.g. if I’d solved equations by balancing, I’d go back and use trial and improvement, then try factorising to solve in a different way…). It seemed like filler at the time, but really I was amassing hours of practice and practising generalising. Our pupils don’t have that time (or inclination…I’d have sooner written two extra pages in silence than whispered to my neighbour). Their teachers have to close that gap by making it explicit.
One of the biggest disservices we do to our pupils is that we leave it up to them to reason and generalise at the point when examples and problems become least familiar. In particular, we disadvantage those who find maths most difficult. It’s scary to show them anything beyond the standard examples, as they become easily confused. It’s also frustrating and slow. But this is why they then can’t generalise: we didn’t show them anything non-standard or, if we did, it was in an exercise when they were floundering on their own with the least support.

I used to think this was the right way to prepare for a topic:
“What sort of questions do I want them to be able to answer?” and planning backwards from there.
This is too narrow. Instead, the question should be:
“What are all the possible forms this topic can take? What’s everything I understand, implicitly, when I look at those questions? Which things have I reasoned for myself, that I don’t even notice myself figuring out?”
I’ll try to make concrete what I’m describing, as I’m realising how far we still have to go in our department:
In the past few days, I’ve been preparing booklets (i.e. textbook chapters) on algebraic expressions (Year 8) and angles on lines and around points (Year 9). Even simple topics have alarming levels of depth to them if approached from the perspective of
“What if none of this was obvious?”
This is just the examples for coefficients. Imagine a weak pupil. Heck, imagine a normal pupil! Are any of these really obvious?
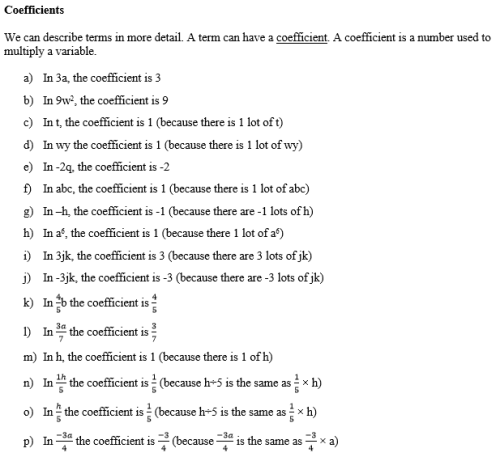
Goodness knows that, until now, I probably only showed them two of these, three if they were lucky. And then I couldn’t understand their inflexible thinking. It will take a long time to go through all of these with my lovely fourth-quartile group, and it will take a lot of pausing, checking and mini-whiteboards. But if not now, when? If I don’t show them now, I am implicitly deciding they aren’t to know it. And, by implication, that top grades aren’t to be an option for them. Top grades might not be realistic, but it shouldn’t be because I didn’t teach them.
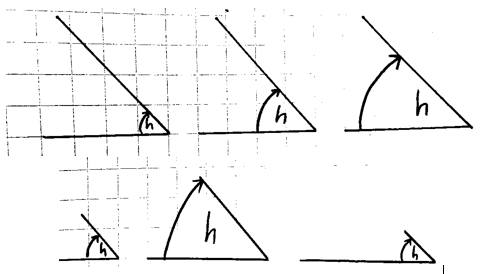
Here is one section – of many more – of the examples to show that angles can look different but be equal. And these aren’t even that different! But I had assumed most of this was obvious before now, or maybe only shown Image A and Image D. D and E as comparisons were thanks to a suggestion from a colleague.
Here are some of the question types I have been playing with just for angles on a line and around a point. And I have a horrible feeling it’s still only scratching the surface. There is so much implicit knowledge in here: matching angles, right angle notation, when and how to use algebra to solve, spotting straight angles in the midst of full turns, spotting two distinct sets of 180 on a single straight line… It is really scary to imagine teaching it. It is even scarier to think that for 6 years I have allowed pupils to walk into exam halls (or worse…the world) where they have no help or explanation the first time they come across it.
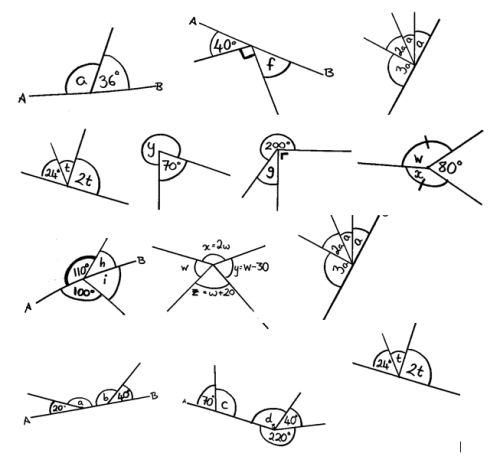
Given the sheer volume of implicit knowledge and modelling needed (especially for the second and third from last….they will melt the minds of all but the most confident. Terrifying!), we’re expecting that ‘angles on lines and around points’ will be at least three lessons.
So far, I think that’s my contribution when people say
“What do you mean by a mastery curriculum?”
I think it might be simplest to summarise as
“We tell them everything we know, even when we didn’t realise we knew it.”
Are you intrigued, enraged or perplexed by what we do? Come visit!
Are you delighted or enthralled? Apply! We’re looking for a teacher for September 2017 (or sooner, for the right candidate). The ad is on TES now, closing SOON: https://www.tes.com/jobs/vacancy/maths-teacher-brent-440947
Get in touch on dquinn [at] mcsbrent.co.uk or [at]danicquinn on Twitter to chat or arrange a time to call in.





